In the realm of physics, collisions present a fascinating study into the behavior of energy and momentum. Specifically, inelastic collisions, which involve the deformation or generation of heat, prompt the question: Is energy conserved? This discourse elucidates the complexities associated with energy transformation during such collisions, with a focus on a crucial principle: while kinetic energy may not be conserved, the total energy, including other forms, remains constant.
To fully appreciate the nuances of energy conservation in inelastic collisions, one must first explore the foundational principles of mechanics and the types of collisions that exist. Understanding the difference between elastic and inelastic collisions is pivotal in grasping the energy dynamics involved.
Understanding Collisions: Elastic vs. Inelastic
Collisions are broadly classified into two categories: elastic and inelastic. The primary distinction rests in the conservation of kinetic energy.
In elastic collisions, both momentum and kinetic energy remain conserved. When two objects collide elastically, they bounce off each other without any permanent deformation or generation of thermal energy. A classic example can be seen in the interaction of billiard balls. Upon impact, they transfer kinetic energy efficiently, maintaining the total energy within the system.
Conversely, inelastic collisions are characterized by a different outcome. During such events, momentum is conserved while kinetic energy is not. Instead of bouncing apart, colliding objects might crumple, stick together, or undergo a permanent change in shape. A mundane example would be a car crash where vehicles crumple upon impact, resulting in throngs of energy dissipated as sound and heat. This scenario typifies the conversion of kinetic energy into other forms of energy, rendering some of it non-recoverable within the system.
The Conservation of Energy: A Broader Perspective
Despite kinetic energy losses, the law of conservation of energy is sacrosanct and unequivocal throughout physics. This principle states that energy cannot be created or destroyed; it can only transform from one form to another. Hence, while kinetic energy may be sacrificed during an inelastic collision, it does not vanish.
During a collision, several energy transformations occur. For instance, kinetic energy is often converted into:
- Heat energy: The friction between surfaces or internal friction within the materials can generate heat. For example, when two cars collide, their deformation and the friction at the point of contact lead to an increase in temperature.
- Sound energy: The noise produced during a collision results from the rapid release of energy. This energy resonates through the air, manifesting as sound waves that propagate outward.
- Potential energy: If the objects involved in the collision undergo vertical movement—such as a ball being dropped—the kinetic energy involved can transition into gravitational potential energy at its peak height before falling back, where it is again converted back to kinetic energy upon descent.
Mathematics of Inelastic Collisions: Analyzing the Impact
To dissect the energy dynamics in an inelastic collision, let’s delve into the mathematics that governs the process. The conservation of momentum can be expressed as:
m₁v₁ + m₂v₂ = (m₁ + m₂)v_f
Here, m₁ and m₂ represent the masses of the colliding bodies, v₁ and v₂ their initial velocities, and v_f the final velocity of the combined mass post-collision.
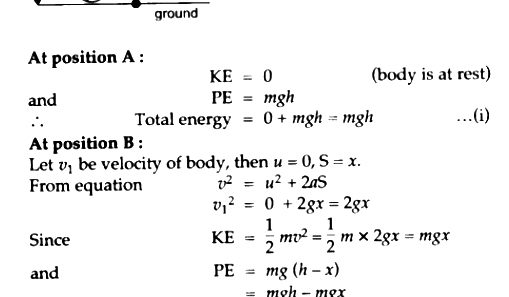
However, kinetic energy before and after the collision will not be equal. The initial kinetic energy can be calculated as:
KE_initial = 0.5 * m₁ * v₁² + 0.5 * m₂ * v₂²
The kinetic energy after the collision, on the other hand, will be represented as:
KE_final = 0.5 * (m₁ + m₂) * v_f²
As evident, for inelastic scenarios, KE_initial will be greater than KE_final. The difference in kinetic energy signifies energy lost to other forms, illustrating the transformation — a fundamental aspect of physics that can be vital in engineering and safety assessments.
Real-World Implications: Safety and Engineering
The principles governing energy transformation during inelastic collisions have profound real-world implications. In automotive engineering, understanding these forces is integral to designing crumple zones in vehicles. These zones are engineered to deform upon impact, dissipating energy and thereby reducing the risk of injury to occupants.
Moreover, investigating inelastic collisions plays a significant role in crash testing and safety standards. Engineers analyze collision data to enhance the resilience of materials used in construction and vehicles. Furthermore, knowledge of energy dissipation helps shape regulations aimed at improving public safety.
The Conclusion: Energy Transformation is Inevitable
Ultimately, the discourse surrounding the conservation of energy in inelastic collisions reveals a complex relationship. While kinetic energy may not be retained post-collision, the entirety of energy remains conserved in different forms. This principle not only underpins fundamental physics but also branches into practical applications that safeguard our daily lives. As we unravel the mysteries of energy dynamics, it becomes clear that energy transformation is an inextricable part of nature, influencing both our understanding of the physical world and our endeavors to design safer systems.