In the realm of classical mechanics, energy conservation stands as a fundamental principle that governs the interactions of physical bodies. Among these interactions, collisions represent a critical area of inquiry, particularly elastic collisions. This article delves into the nature of energy conservation during elastic collisions, exploring the intricate dynamics at play and addressing key uncertainties surrounding this phenomenon.
At the heart of the discussion lies the definition of elastic collisions. These are interactions where both momentum and kinetic energy are conserved. Unlike inelastic collisions, where some energy is transformed into other forms—such as thermal energy or sound—elastic collisions maintain the original kinetic energy of the system. This unique characteristic invites contemplation into how energy conserves itself as objects collide, rebound, and ultimately part ways.
Understanding elastic collisions requires an examination of the conditions under which they occur. For instance, ideal conditions for elastic collisions often manifest in microscale interactions, such as atomic or molecular collisions, where the magnitude of external forces is negligible. In practice, however, macroscopic collisions—like those seen in sports or vehicular impacts—tend toward inelastic behavior, primarily due to the dissipation of energy through deformation, heat, and sound. Thus, while the principles of elasticity serve as a robust theoretical foundation, real-world applications frequently deviate from this ideal.
To appreciate the behavior of energy in elastic collisions more profoundly, one must consider two key principles: the conservation of momentum and the conservation of kinetic energy. These principles operate in concert to illuminate the behavior of colliding bodies.
In the context of conservation of momentum, the total momentum of a closed system before and after a collision remains constant. Mathematically expressed as ( p_{initial} = p_{final} ), this principle applies universally, whether the collision is elastic or inelastic. By analyzing the momentum vectors of colliding objects, one can predict outcomes, such as their velocities post-collision, contingent upon their masses and initial velocities.
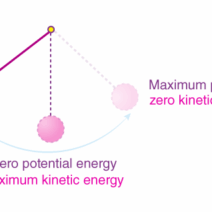
Conversely, conservation of kinetic energy in elastic collisions asserts that the total kinetic energy before and after the collision remains unchanged. This is encapsulated in the formula ( KE_{initial} = KE_{final} ). To elaborate, if two billiard balls collide elastically, the total kinetic energy prior to impact will equate to the total kinetic energy after the impact, assuming no external influences interfere. Such precision in energy conservation demystifies the fleeting yet fascinating collisions that occur in our everyday lives.
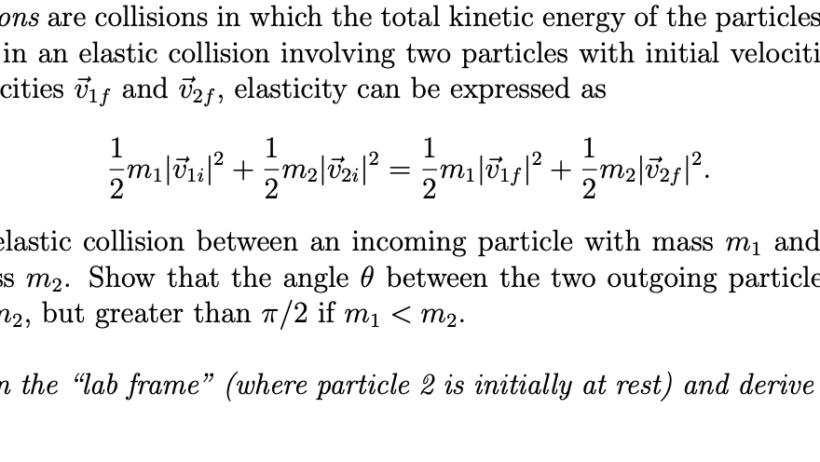
Let us now delve deeply into the mathematical treatment of perfectly elastic collisions, which hinges on an understanding of the velocities of colliding bodies. When two objects of different masses collide elastically, their velocities can be expressed using the equations:
For object 1:
( v_{1f} = frac{(m_1 – m_2)v_{1i} + 2m_2v_{2i}}{m_1 + m_2} )
For object 2:
( v_{2f} = frac{(m_2 – m_1)v_{2i} + 2m_1v_{1i}}{m_1 + m_2} )
Here, ( m_1 ) and ( m_2 ) denote the masses, while ( v_{1i} ) and ( v_{2i} ) are the initial velocities. These equations elegantly illustrate how the velocities redistribute upon collision, ensuring that while kinetic energy may be transformed between the colliding entities, the total energy remains invariant—a linchpin for understanding energy dynamics in elastic collisions.
However, realistic scenarios often question the application’s integrity of such models. The ongoing inquiry includes whether absolute, perfect elastic collisions can ever truly exist. Factors like surface roughness, internal energy losses, and external forces invariably complicate the assertion of energy conservation. For example, consider two superballs colliding in an ideal environment. While they may seemingly represent perfect elasticity, microscopic irregularities and energy dissipation upon contact remind us of the complexities embedded in physical reality.
In addressing potential concerns regarding energy conservation in elastic collisions, we must explore scenarios wherein energy does not appear conserved at first glance. When dealing with angular momentum or rotational dynamics, the conservation laws may convolute the perceived energy balance. Here, examining the coefficient of restitution, a measure of elasticity, provides clarity. It quantifies how much kinetic energy remains post-collision, offering insight into transitional states between elastic and inelastic collisions. A coefficient of one signifies perfect elasticity, while values less than one indicate energy loss—and thus a departure from pure elastic behavior.
The investigation into elastic collisions is fundamentally a reflection of nature’s complexity. In every interaction, we uncover layers of energy transformation, loss, and conservation, offering a window into fundamental physical laws. This interdisciplinary interaction among physics, engineering, and even philosophy fosters a richer understanding of not only collisions but of the universe’s underlying principles. It compels us to appreciate the highly nuanced dance of energy as it flows seamlessly from one form to another, perpetually guided by the laws that govern the physical world.
In summation, while energy conservation holds steadfast in elastic collisions at a theoretical level, real-world applications often reveal a tapestry woven with complexity. Distinguishing between pure elastic interactions and real-world implications continues to challenge our understanding. Nonetheless, the elegance and predictability inherent in ideal elastic collisions serve as a powerful reminder of nature’s coherence, urging continual exploration and curiosity at the intersection of energy and motion.