Mechanical energy is a crucial concept in physics, embodying the sum of kinetic and potential energy in a system. The notion of whether mechanical energy is conserved across various systems invites rigorous examination. Understanding this principle is foundational for students of physics, engineers, and environmental scientists, as it plays a pivotal role in analyzing energy transformations and system behaviors. This article embarks on an exploration of the conservation of mechanical energy, elucidating the underlying principles, the conditions that govern energy conservation, and specific applications across diverse systems.
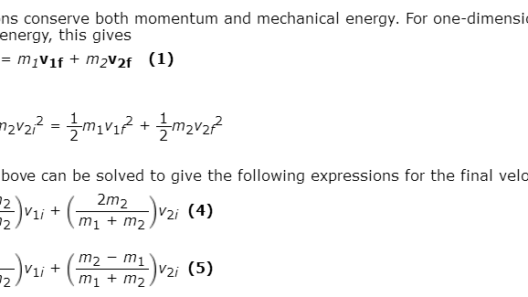
Conservation of mechanical energy predominantly asserts that in a closed system devoid of non-conservative forces, the total mechanical energy remains constant. The interplay between kinetic energy (energy of motion) and potential energy (stored energy) characterizes this phenomenon. A myriad of systems exemplifies this principle, yet complexities arise when non-conservative forces, such as friction or air resistance, intervene.
Exploring key components of mechanical energy conservation illuminates its application in various contexts. The conditions under which mechanical energy is conserved depend significantly on the nature of the forces acting on the system. Examining conservative and non-conservative forces enhances comprehension of energy transformations.
Understanding Conservative Forces
Conservative forces are defined by their unique property: the work they perform on an object is independent of the path taken during the movement. Instead, it relates solely to the initial and final positions of the object. Gravitational force and elastic spring force serve as quintessential examples of conservative forces. When an object moves within a gravitational field, the potential energy it acquires depends solely on its height relative to a reference point, not the trajectory taken.
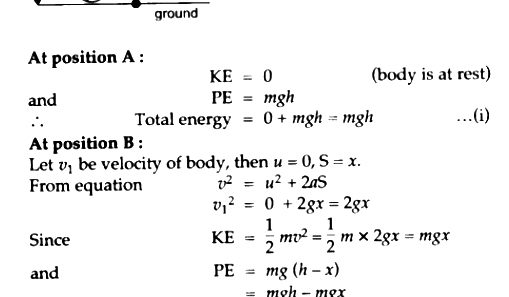
In a frictionless environment, where only conservative forces operate, mechanical energy remains conserved. An illustrative case can be derived from the mechanics of a pendulum. As the pendulum swings, it continually converts kinetic energy into potential energy and vice versa. At the apex of its swing, the pendulum momentarily halts, embodying maximum potential energy and minimal kinetic energy. Conversely, at the lowest point, it achieves peak kinetic energy, corresponding to diminished potential energy. Throughout these oscillations, the total mechanical energy persists consistently—the art of conservation in motion.
Dynamics of Non-Conservative Forces
Contrarily, non-conservative forces are dependent on the path taken and are often responsible for energy dissipation within a system. Friction, air resistance, and other dissipative forces convert mechanical energy into thermal energy, thereby reducing the total mechanical energy available for performing work. This depletion marks the crucial difference between conservative and non-conservative interactions.
In the context of mechanical energy conservation, systems subjected to non-conservative forces present complexities. For instance, consider a block sliding down a rough incline. As the block accelerates due to gravity, it encounters frictional forces that act against its motion. The gravitational potential energy translates into kinetic energy, yet part of this energy dissipates as heat due to friction, resulting in lower overall mechanical energy at the bottom of the incline. Herein lies the essential realization: while mechanical energy may not be conserved, the total energy of the system—consisting of mechanical and thermal energy—still adheres to the conservation law in a broader perspective.
Applications of Mechanical Energy Conservation
In practical applications, the principles of mechanical energy conservation find extensive utility in fields ranging from engineering to environmental science. The design of roller coasters provides a vivid demonstration of energy transformation principles. By harnessing gravitational potential energy as the coaster ascends to loftier heights, the design subsequently allows for kinetic energy transformation in the descent. The interplay of forces and energy conversions in such structures reveals the intricacies of mechanical energy conservation even amidst losses dictated by non-conservative forces.
Similarly, in environmental engineering, understanding mechanical energy conservation becomes substantial for optimizing systems such as hydroelectric dams. The principle of converting the potential energy stored in elevated water into kinetic energy driving turbines underscores how conservation principles govern efficiency in energy generation. Design efforts focus on minimizing friction losses and maximizing energy efficiency, highlighting the importance of maintaining mechanical energy to enhance energy output.
Challenges in Real-World Systems
Despite the foregone conclusions of energy conservation laws, real-world applications often confront challenges that complicate mechanical energy conservation. System inefficiencies, including friction, air drag, and other dissipative phenomena, frequently challenge the attainment of ideal conservation conditions. Moreover, systemic boundaries and external influences can alter energy dynamics, necessitating ongoing research to optimize systems for efficiency and performance.
In summary, the nebula of mechanical energy conservation is intricate, rooted in the balance of kinetic and potential energies. While ideal scenarios uphold the principle of conservation in closed systems dominated by conservative forces, practical realities often introduce complexities necessitating deeper considerations. Recognizing the influence of non-conservative forces provides insights that enhance our understanding of energy dynamics throughout a diverse spectrum of systems. Ultimately, this exploration fosters an appreciation for the mechanics of energy as an omnipresent, transformative force in our world.