The interplay of energy transformations is one of the fundamental principles that defines the physical world. At the core of this intricate dance lies the Law of Conservation of Energy, a monumental concept that asserts energy is neither created nor destroyed; it merely changes forms. This principle manifests in myriad systems—from the orbits of celestial bodies to the oscillations within a guitar string. Central to this law is a mathematical equation that encapsulates its essence.
Energy conservation is often articulated through a concise representation that is remarkably elegant: E_initial = E_final. This equation serves as a graphical depiction of an idea so profound that it transcends the realms of physics, chemistry, and biology. Understanding this equation is crucial to comprehending the multitude of processes that underlie our universe. Let us delve deeper into the mathematical expression and the corollaries it entails.
The energy involved in any system can generally be categorized into two forms: kinetic energy and potential energy. These forms can interchange under varying conditions, but their sum within an isolated system remains constant. The beauty of this law lies in its universal applicability; whether we are examining an elegant swan gliding across a pond or the dramatic collision of celestial bodies, the conservation of energy is the underlying truth that governs these phenomena.
The Intricacies of Energy Types
To fully grasp the mathematical expression of energy conservation, one must explore the types of energy involved in mechanical systems.
Kinetic Energy is the energy of motion. Any object that moves possesses kinetic energy, which is mathematically defined as:
K.E. = 1/2 mv²
Here, m represents the mass of the object and v represents its velocity. This equation exemplifies the quadratic relationship between velocity and kinetic energy; a small increase in the speed of an object results in a significant increase in its kinetic energy.
Potential Energy is stored energy based on an object’s position or arrangement. For gravity-associated contexts, potential energy can be expressed as:
P.E. = mgh
In this equation, m is the mass, g is the acceleration due to gravity, and h is the height above a reference point. This reveals another layer of complexity—potential energy increases with height, implying that even stationary objects possess energy waiting to be unleashed.
The amalgamation of these two energy types leads us to the equation that embodies the Law of Conservation of Energy:
K.E._initial + P.E._initial = K.E._final + P.E._final
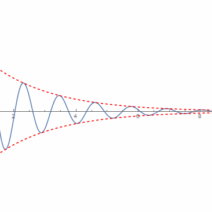
This relationship confirms that the total energy before a transformation is equal to the total energy after the transformation. In simpler manifestations, this might be observed in a pendulum swinging back and forth, where energy oscillates between kinetic and potential forms, but the total energy remains consistent throughout its motion.
Visualizing Energy Conservation Through Practical Examples
Let us explore the implications of this equation in both simple and complex systems.
Consider a child on a swing. At the highest point in the swing’s arc, the child has maximum potential energy and minimal kinetic energy. As the swing descends, potential energy is converted into kinetic energy, culminating in peak speed at the lowest point, where kinetic energy is maximized. As the swing ascends to the opposite height, kinetic energy dissipates into potential energy once more. This cyclical behavior is not merely a delightful playground observation; it is a tangible illustration of the conservation of energy at work.
Now, zooming out to a grander scale, the dynamics of planetary motion further illustrate this law. The gravitational pull between Earth and the moon creates a system where gravitational potential energy and kinetic energy are in constant flux. As the moon orbits, it exchanges potential energy for kinetic energy and vice versa, holding steadfast to the principle that, overall, energy remains conserved in this cosmic dance.
Embracing the Implications of Energy Conservation
The ramifications of the Law of Conservation of Energy ripple across diverse fields, influencing not only physical sciences but also engineering, environmental science, and renewable energy initiatives.
In engineering, this principle is pivotal in the design of more efficient systems. By recognizing that energy must be conserved, engineers can innovate designs that minimize energy waste, promoting sustainability.
In environmental science, understanding energy conservation is essential for assessing ecological dynamics. For instance, in ecosystems, energy from the sun is captured by plants and transferred through food webs, adhering to the conservation law. This comprehension informs policies aimed at preserving biodiversity and mitigating climate change.
Moreover, the proliferation of renewable energy technologies—such as solar panels and wind turbines—is intimately linked to the conservation of energy principles. Harnessing natural energy forms, converting and storing them efficiently reflects our desire to work in harmony with nature while adhering to immutable physical laws.
The Law of Conservation of Energy is not merely an abstract principle; it is a profound, underlying truth that resonates through every interaction in our universe. As we harness this knowledge, we can foster a deeper appreciation for the delicate balance of energy forms surrounding us, prompting innovative solutions toward a more sustainable future.